Dopo il successo dell’evento «Old and New trends in Mathematical Collaboration between Brazil and Italy» (https://sites.google.com/view/indamwrome25/homeAC), celebratosi a Roma dal 23 al 27 Giugno del 2025, durante il quale è stato siglato uno storico Memorandum of Understanding (MoU) tra il prestigioso “Istituto Nazionale di Matematica pura e Applicata” di Rio de Janeiro e l’Istituto Nazionale di Alta Matematica “Francesco Severi” a Roma, ecco servito, quasi su un piatto d’argento, un altro esempio di come la matematica, questa temuta sconosciuta, possa fungere da potente veicolo di diplomazia scientifica.

       Le notizie di rilievo, in tal senso, sono almeno due. La prima è che, dopo una pausa di circa due anni, la London Mathematical Society (LMS) ha rilanciato nel mese di Novembre del 2024 il già ampiamente collaudato programma Mentoring African Research in Mathematics (LMS-MARM).  La seconda è che il 21 Luglio del 2025, presso l’Università di Lubumbashi, in Repubblica Democratica del Congo, è stata inaugurata la prima edizione delle Lezioni “Luca Attanasio e Vittorio Iacovacci” per la formazione matematica dei giovani ricercatori, affiancate dai laboratori “Mustapha Milambo” per il consolidamento pratico della teoria (https://unilu.lms-marm-africa.org/).

         Il filo che unisce le due notizie è che le predette lezioni, istituite in via permanente alla memoria dell’Ambasciatore d’Italia a Kinshasa Luca Attanasio, caduto il 22 febbraio 2021 in un attentato a Goma insieme al carabiniere Vittorio Iacovacci e all’autista del convoglio ONU che li trasportava, Mustapha Milambo, si sono tenute proprio all’interno della prestigiosa cornice del programma LMS-MARM. Che funziona in maniera semplice ed efficace.  Il consiglio direttivo, fino ad oggi guidato dal professor Frank Neumann dell’Università di Pavia, designa, all’inizio di ogni nuovo ciclo, quattro mentor allo scopo di affiancare nell’attività di ricerca matematica giovani studiosi affiliati ad altrettante università africane. L’incarico ha durata annuale ed è rinnovabile, previa valutazione positiva, per un ulteriore periodo di dodici mesi. Si tratta del termine entro il quale ci si attende un consolidamento di stabili collaborazioni scientifiche con le istituzioni africane coinvolte dal programma, quale indicatore misurabile della sostenibilità dell’intervento.

         Attivo da circa vent’anni,  il programma MARM ha già promosso, attraverso l’erogazione di appropriati contributi, collaborazioni con un ampio ventaglio di nazioni africane. Tra queste vale la pena di ricordarne alcune che figurano nella lista dei “paesi pilota”, come tali individuati dal Piano Mattei, quali  il Marocco, l’Etiopia, la Repubblica del Congo, il Kenya, la Costa d’Avorio, la Tanzania, il Ghana e il Senegal. Il settimo ciclo, tutt’ora in corso, ha attuato collaborazioni con i dipartimenti di matematica dell’École Normale Superieur in Gabon, dell’Università di Makurdi in Nigeria,  dell’Università di Dodona, in Tanzania e, per la prima volta, come anticipato, con quello dell’Università di Lubumbashi (UniLu), nella Repubblica Democratica del Congo, teatro del riuscitissimo esperimento di diplomazia scientifica ricordato in apertura, che si è svolto tra il 20 Luglio e il 1° Agosto.  Le attività di addestramento alla ricerca, spalmate lungo l’arco di dieci giorni, hanno visto l’alternarsi di lezioni monografiche a sedute di problem solving. Quest’ultime sono culminate con il workshop «Aujord’hui on parle de Matématiques», realizzato nel contesto dei laboratori Mustapha Milambo, diretti dal professor Franck Kalala Mutombo, già autorevole direttore scientifico dell’African Institute for Mathematical Science, con sede in Senegal, e sostenuti dal direttore del dipartimento di Matematica, Professor Kumwimba Seya Didier. La peculiarità del workshop è consistita nell’affidare l’esposizione dei  seminari  esclusivamente ai giovani partecipanti, opportunità che questi ultimi hanno accolto con incontenibile e contagioso entusiasmo. Un ulteriore esempio, in aggiunta di altri che potrebbero farsi, sufficiente a destituire di fondamento ogni banale pregiudizio secondo cui  l’attuazione in Africa di uno qualsiasi di questi progetti di cooperazione matematica, vuoi della London Mathematical Society o vuoi dell’Unione Matematica Internazionale, equivalga all’insegnamento di argomenti elementari ad un capitale umano per lo più  inesperto.

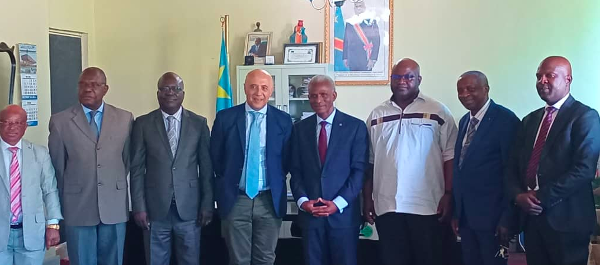
L’Ambasciatore Dino Sorrentino tra il Rettore Gilbert Kishiba Fitula (alla sua sinistra) e il Prorettore, Jean-Marie Dikanga Kazadi (alla sua destra). Alla sinistra del Rettore, Franck Kalala Mutombo.

La locandina dell’evento

Al contrario, la visita a Lubumbashi è stata, per chi scrive, un’esperienza di grandissimo valore  non solo umano ma soprattutto scientifico. Una gradita sorpresa quella di incontrare studenti di talento e profondamente motivati, animati da una irresistibile sete di conoscenza matematica accompagnata anche da una grande padronanza delle buone maniere. A titolo esemplificativo, in ogni circostanza ufficiale, essi sono abituati a rivolgersi gli uni agli altri usando quelle formule di cortesia ormai così rare in Europa (dove ci si dà tutti del tu), come l’uso del Monsieur, del Madame o del pronome «vous(il “lei”).  Se a tutto ciò si aggiunge una spruzzata di solida preparazione di base, per certi versi inattesa, non c’è da stupirsi che lezioni di durata programmata di novanta minuti si dilatassero fino a tre o quattro ore, mutandosi in discussioni vivaci, condotte con rigore e competenza ma anche con naturalezza e la stessa leggerezza e «amusement» con  cui si sarebbe potuto parlare di calcio al bar dello sport.   E’ vero che è più raro imbattersi, oggidì, in giovani così motivati negli atenei europei dotati di eccellenti infrastrutture, o è solo l’impressione di chi scrive? E se sì, perché?

         Lasciando la risposta dell’interrogativo alla sensibilità e/o all’esperienza delle lettrici e dei lettori, è doveroso segnalare che le intense attività matematiche sviluppate nell’ateneo congolese sotto l’egida dell’LMS-MARM, si sono concluse con una importante cerimonia di chiusura, a cui tutta l’Università (e non solo l’area scientifica) è stata chiamata a partecipare. In una raccolta atmosfera di ufficialità,  il Magnifico Rettore dell’Unilu, Gilbert Kishiba Fitula, alla presenza di Walter Noca, Console Onorario italiano a Lubumbashi, ha solennemente comunicato la decisione delle autorità accademiche di intitolare un’aula dell’UniLu (che nel 2025 ha celebrato il settantesimo anniversario dalla fondazione) alla memoria dell’Ambasciatore Attanasio e del Carabiniere Iacovacci. Eleggendola, nel contempo, quale teatro delle future prossime edizioni delle lezioni ad essi intitolate.

         Tale determinazione costituisce un importante tributo alla cultura diplomatica italiana per l’ottenimento del quale hanno concorso una molteplicità di attori e circostanze. Da una parte l’inesauribile energia del professor Kalala Mutombo, coadiuvato dal direttore del dipartimento, professor Didier, con il sostegno di tutte le componenti accademiche dell’UniLu, non solo del Rettore, ma anche anche del Prorettore Jean-Marie Dikanga Kazadi e del Preside della Facoltà di Scienze Assumani Salimini. Dall’altra l’appoggio convinto del Consolato Onorario a Lubumbashi, che ha permesso il raggiungimento di un risultato che, comunque, non sarebbe stato possibile senza il cruciale e generoso appoggio delll’Ambasciatore d’Italia  a Kinshasa, Dino Sorrentino. Egli ha infatti monitorato e sostenuto l’iniziativa sin dalle fasi iniziali, autorizzandone fin da subito il patrocinio da parte dell’Ambasciata. E non sono i soli importanti attori che hanno concorso alla realizzazione della magica atmosfera umana e scientifica delle lezioni “Attanasio e Iacovacci”:  oltre alla LMS,  non si può non menzionare un ruolo da protagonista da parte dell’International Mathematics Master (IMM), con sede a Trieste, da anni impegnata con programmi di educazione matematica in Africa e il supporto della Casa degli Italiani, presieduta dalla Signora Romy Muhemedi Kasongo.

Questi i fatti del progetto LMS-MARM a Lubumbashi. Una domanda sorge però spontanea. Perché? Più precisamente: la matematica può davvero contribuire allo sviluppo economico e sociale del continente africano? In che modo?

         Cominciamo col dire che sarebbe impreciso riferirsi alle nazioni africane come a paesi in via di sviluppo piuttosto che pensarle, invece, quali veri e propri laboratori di economie emergenti. Il punto è che l’Africa non è un continente povero, bensì una terra con tanti poveri. Il progresso economico o tecnologico delle sue nazioni più progredite, si pensi al Sudafrica, ma anche al Kenya, al Rwanda o al Senegal, convive con una miseria diffusa tra milioni di persone. Lodevoli sono le iniziative di numerose ONG o di ricche fondazioni filantropiche (tra cui spicca la Pupkewitz in Namibia e la AMKA, con sede a Roma, nella Repubblica Democratica del Congo), la  maggior parte delle quali opera a mo’ di pronto soccorso, per far fronte alle prime e più urgenti necessità. Fornendo cibo o acqua, per esempio, a popolazioni residenti in aree in cui il loro approvvigionamento è particolarmente difficile e/o in  porzioni di territorio neppure raggiunte dalla rete elettrica. In cui, insomma, manca davvero tutto. Molte delle azioni messe in campo, tutte lodevoli senza eccezioni, sono spesso di natura una tantum, con poche ragionevoli prospettive di innescare un cambiamento strutturale. Si tratta, insomma, di piccole e poche gocce in un oceano di necessità, che possono persino far fronte a situazioni urgenti di sopravvivenza ma non delineano alcuna visione di futuro sostenibile.

         A questo punto, la risposta alla domanda, perché?, non è difficile. E non può che rievocare l’iconica figura del già menzionato Luca Attanasio che, concedendo un’intervista al termine di una delle sue visite presso la Fondazione Amore e Libertà a Kinshasa, affermò: «La comunità sta accompagnando in una zona poverissima della società, delle persone che chiedono una cosa importante, chiedono di poter avere una formazione». Per Attanasio, insomma, la formazione è la chiave per combattere la povertà in modo strutturale. Unica via, se una ce n’è, per offrire, soprattutto ai giovani, l’opportunità di poter plasmare il proprio futuro. Un processo lento, questo, che può richiedere anche varie decine di anni, ma il cui sentiero va intrapreso subito, senza indugi,  con pazienza e determinazione, poiché i grandi cambiamenti richiedono tempi significativamente lunghi. Proprio come la crescita dell’essere umano che, nei primi anni di vita, senza la protezione dei genitori, è in balia delle forze della natura e diventa adulto solo in prossimità dei vent’anni. L’esperienza della storia e la storia dell’esperienza suggeriscono che la crescita di una società, di una comunità di individui, anche in termini di coscienza e di autodeterminazione collettiva, non può esimersi dall’obbedire a simili principi di lenta gradualità.

         Una volta, però, che si sia stipulato, seguendo Attanasio, di attribuire alla formazione un ruolo decisivo per la lotta alla povertà e alle disuguaglianze, perché la più urgente sarebbe quella matematica? Per quale ragione si dovrebbe investire nella ricerca in una disciplina (la cui natura è ignota ai più)  l’importanza  della quale potrebbe apparire risibile se paragonata alla fisica, all’ingegneria, alla medicina o, per dirla in modo ancora più brutale, alle scienze agrarie? La risposta, purtroppo, non è solo quella, ovvia, che la matematica è alla base di tutti i campi dello scibile, una frase che, per chi scrive, non ha neppure molto senso. Certo, il dubbio che il potenziamento della ricerca matematica possa somigliare ad una preoccupazione da “ricchi”, che non necessitano di cimentarsi con la lotta quotidiana per la sopravvivenza, sorge.  E’ però vero il contrario. La supremazia strategica della matematica, rispetto ad altre pratiche scientifiche, è che essa è facilmente implementabile in economie poco sviluppate, con limitate, se non inesistenti, risorse finanziarie. Detta in modo più brutale,  la matematica costa poco. E’ un kit di primo intervento, che non fornisce spaziose sale operatorie attrezzate a puntino (laboratori di fisica, chimica o ingegneria), bensì una cassetta del pronto soccorso, con rimedi semplici, non costosi ma la cui efficacia può essere risolutiva.

         Tale intuizione di fondo è fatta propria anche dalle Nazioni Unite, soprattutto nell’enunciazione dei 17 Sustainable Development Goals. L’esortazione di base è quella di promuovere la cultura STEM nelle economie emergenti. STEM è l’acronimo di Science, Technology, Engineering e Mathematics. Mathematics è l’utima parola chiave dell’acronimo, quasi come se essa non dovesse essere inclusa nella Science della sua prima lettera. La ragione è, tuttavia, che se occorrono capitali e finanziamenti per attuare programmi tecnologico/ingegneristico di primo livello,  per la matematica basta poco. Una lavagna, dei fogli di carta, voglia di pensare. Una connessione internet, forse, ma è praticamente tutto. In cambio di cosa?

         Diciamola tutta, allora. Il nostro PC, così come lo conosciamo, è il risultato dei primi studi puramente teorici, di natura puramente teorica, di teoria della computabilità ideata dal matematico Turing, il medesimo che scongiurò l’attacco aereo nazista su Londra. Si obietterà che siamo già nell’era dei supercalcolatori quantistici, i quali rivoluzioneranno, come già hanno cominciato a fare, il nostro modo di interfacciarci con la macchina. Scopriremmo, però, che il «quantum computing» è anch’essa una teoria matematica puramente teorica. Cioè.  Alla portata di tutte le menti brillanti, indipendentemente dal censo e, quindi, anche di quelle delle società più povere, purché le si sappia riconoscere ed orientare. Stesso discorso vale per le scienze  dei Big Data, delle reti neurali, persino dell’intelligenza artificiale (ecco un link per il lettore scettico: https://arxiv.org/abs/2501.10465), dell’apprendimento automatico. Invero, tutti gli ultimi ritrovati della tecnologia nascono da ricerche di matematica pura. In altri termini,  il potenziamento della ricerca matematica, nel quand’anche debole tessuto educativo delle economie emergenti, è in grado di allargare drammaticamente la platea dei detentori di know-how tecnologico, rendendosi potenzialmente interessanti per imprese straniere che si disporranno a collaborare alla pari,  fornendo capitali per realizzare infrastrutture progettate e/o gestite con competenza da energie intellettuali locali. La matematica, insomma è,  questa è la tesi,  il software necessario per lo sviluppo dell’hardware tecnologico. Attenzione, non la si vuole spacciare come un magico toccasana, un sostituto delle infrastrutture tecnologiche necessarie a sviluppare tutte le predette pratiche. Si può addestrare, e di fatto ciò è già in essere in varie nazioni, come Kenya e Sudafrica, per esempio,  un capitale umano all’uso di tali strumenti pur senza che questo disponga di una specifica competenza matematica o pur, esagerando, essendo totalmente ignaro di essa. Tuttavia, ciò condannerebbe le società beneficiarie di tali tecnologie ad un ruolo di subalternità poiché, se l’implementazione dell’esistente può prescindere da una consapevolezza matematica, la sua innovazione solo sarebbe possibile attraverso la diffusione di una sostanziosa cultura matematica.  Ed è ciò che costituisce il principio ispiratore dell’importante African Institute of Mathematical Science che possiede centri in Sudafrica, Senegal, Ghana, Rwanda e Camerun, e promuove master e ricerca in matematica applicata nella certezza che sia il primo immediato cammino per l’ammodernamento delle giovani società africane.

         Ultimo, ma non meno importante, aspetto da mettere in evidenza è che, sebbene la Repubblica Democratica del Congo non figuri (ancora) nella lista dei Paesi Pilota del Piano Mattei, la Regione Piemonte ha incoraggiato l’esposizione del proprio brand per le lezioni “Attanasio e Iacovacci”, per tramite dell’Assessore alla Cooperazione Maurizio Marrone, che nelle stesse ha ravvisato una indubitabile aderenza ai principi ispiratori del Piano Mattei, il quale pone l’istruzione e la formazione tra le sue sei direttrici fondamentali di intervento, al fine di favorire uno sviluppo sostenibile e «non predatorio» nel continente africano (si veda per esempio https://www.mur.gov.it/it/piano-mattei-ricerca-e-alta-formazione).

Le Lezioni “Luca Attanasio e Vittorio Iacovacci” sono dunque un esempio pilota di come rendere operativo il Piano dal basso. Progettando e implementando una proposta formativa concreta in grado di valorizzare capacità locali per mezzo del perfezionamento dei giovani ricercatori. Il supporto morale dell’Ambasciata d’Italia, visibile attraverso il ricordato patrocino e la sua presenza più che simbolica, si allinea pienamente alle linee guida del Piano, che contempla il coinvolgimento di rappresentanze diplomatiche come parte attiva del “Sistema-Paese” italiano. I Laboratori Mustapha Milambo, pensati per addestrare alla pratica delle nozioni apprese, per incoraggiare approfondimenti e per promuovere seminari organizzati e tenuti da giovani potenziali ricercatori, come nel già ricordato “Aujourd’hui on parle de mathématiques”, ha dato voce e visibilità alle riflessioni e il talento dei giovani partecipanti, incarnando il tipo di formazione integrata, interattiva e accademicamente robusta che il Piano Mattei prevede per promuovere uno sviluppo locale concreto, replicabile e sostenibile.

         Per questo le lezioni Attanasio e Iacovacci, coi Laboratori Milambo, sono state non solo un importante esperimento scientifico didattico, ma anche un esempio di come i piani, che possono essere condivisi o meno,  criticati o migliorati, non vedranno mai alcuna attuazione se non si prova ad impegnarsi, al di là delle sfumature di vedute, a farli funzionare così, in prima persona, anche in piccole realtà e con piccoli passi. Come a Lubumbashi, banco di prova e teatro di applicazione sperimentale dei principi del Piano, senza che essa sia città di un Paese Pilota. Sulla carta.

Letterio Gatto

docente di Algebra Lineare e Geometria

Politecnico di Torino

Quasi fosse una parola magica in grado di mutare, come la pietra filosofale, ogni vile metallo in oro, l’acronimo STEM, ormai massicciamente usato anche nel nostro italico idioma, viene evocato in pressoché tutti i documenti emessi da importanti istituzioni internazionali (quali le Nazioni Unite con l’Agenda Vision 2030 per lo sviluppo sostenibile) a cui, almeno sulla carta, stiano a cuore le tematiche della cooperazione con i paesi, cosiddetti, in via di sviluppo.

Per chi non lo ricordasse,  STEM sta per Science, Tecnhology, Engineering e Mathematics, un quadrinomio che ha tutto il piglio della via maestra che presiede all’elaborazione di progetti di sviluppo solidi e sostenibili.  E’ anche latore, però, di  un messaggio che, a volerlo leggere attentamente, non può non suscitare curiosità e attenzione. Che la prima lettera dell’acronimo stia per Scienza va bene, è ragionevole, ci mancherebbe altro. Un po’ vago, magari o, eventualmente, troppo onnicomprensivo.  La T di Tecnologia, anch’essa imprescindibile,  sembra tuttavia voler fare il paio con la E di ingegneria, suggerendo un non so che di ridondante, non foss’altro per una approssimativa tendenza ad assimilare l’una all’altra, dato che la tecnologia attinge alll’ingegneria e questa da quella in una mutua simbiosi la cui efficacia, nel bene o nel male, è sotto gli occhi di tutti. Sorprende quindi che, a fronte di una certa qual vaghezza di significato delle prime tre iniziali, l’ultima di STEM, la M,  indichi con accurata precisione la  Matematica. Si badi. Non fisica, non  biologia o non, persino, medicina che, forse, anzi sicuramente,  già sono state pensate, e riassunte, nel più onnicomprensivo Scienza. Bensì matematica.  Che pure scienza è, ma che è richiamata quasi come se estratta dalla sua propria nicchia, fuori dalla repubblica popolata dalle proprietarie delle prime tre iniziali, come per distinguerne un ruolo che vuol sapere molto più di strategia piuttosto che di quella tattica spicciola con cui ci si illude di  poter fomentare l’innovazione con interventi calibrati sul breve periodo.

Tutto ciò non è casuale, naturalmente,  e non solo perché,  come si dice, “la Matematica è dappertutto”,  un pur innegabile dato di fatto. Dai codici a barre a quelli QR  (felici applicazioni della sofisticata teoria dell’omologia persistente), ai PIN delle nostre carte bancarie, dalla teoria dei Big Data agli studi sulla formazione del consenso, la matematica ha invaso ogni piega della vita delle donne e uomini moderni. Per non parlare, ancora,  dei motori di ricerca che rendono così agevole, al modico prezzo di cessione di risibili, si fa per dire, porzioni della nostra “privacy”, l’accesso ai vari contenuti della rete che, già da una ventina d’anni, è divenuta un  “sistema complesso” nel suo più rigoroso senso matematico.

C’è dell’altro, però, su cui potrebbe ragionarsi, a proposito della M di STEM.  Qualcosa certamente non sfuggito agli studiosi o politici impegnati a confezionare ricette di ripresa economica e progresso sociale.

Primo, la matematica è, tra tutte le scienze, quella più affine alle discipline, cosiddette, umanistiche, come provano decine e decine di esempi di grandi scrittori evidentemente soggiogati dal suo fascino. E non occorre risalire a Dante, col suo Euclide Geomètra tra gli Spiriti Magni del Nobile Castello del Limbo. Basti pensare alle immortali pagine sulla geometria non euclidea dei “Fratelli Karamazov” di Dostoeevski. O all’uomo senza qualità di  Robert Musil. il cui protagonista matematico altro non è che un  “Giovane Torless”, l’adolescente sbalordito dai numeri complessi, che, giunto a maturità, lascia che l’entusiasmo ceda il passo ad una osservazione disillusa del mondo. Si può ancora pensare al Borges della Biblioteca di Babele, o al Joyce nelle ultime pagine del capolavoro Dedalus che, artificialmente, conclude una lista che potrebbe naturalmente allungarsi.

Secondo,  a fronte dell’altissima qualità in termini di contributi alla formazione di know-how e di stimolo all’innovazione tecnologica, la Matematica è la disciplina che può essere praticata più a buon mercato. Alla stragrande maggioranza dei matematici professionisti, e inizianti!, non occorre nulla più che un foglio di carta e una penna o una lavagna e un gesso. A supporto di tale tesi, si legga per esempio “La ruota e il ruotino: perche la didattica a distanza non è la soluzione”, di Andrea Ricolfi, il cui grido di allarme e di avviso si leva per scongiurare l’abiura della tradizionale pratica in favore del “digitale è bello”, cavalcato maldestramente durante la pandemia.

La Matematica, per farla breve, non richiede  gli investimenti necessari per allestire una stazione spaziale o fabbricare un acceleratore di particelle.  Al tempo stesso è irrinunciabile. Non si otterrebbero risultati migliori, in un’economia giovane, preferendole un prodotto più caro e più sofisticato, tanto  essa  già finemente lo è, senza rinunciare ad essere “popolare”, cioè a buon mercato, come era ieri, come è oggi e come sarà domani.

Di qui l’importanza che, in via teorica, si attribuisce allo sviluppo della cultura matematica  per la crescita delle economie giovani come, per esempio, quelle dell’Africa subequatoriale che, tra l’altro, non hanno ancora scongiurato il rischio di vedersi catapultare in una crisi alimentare creata artificialmente, innescata da un conflitto, quello Russo-Ucraino, che pur si consuma in aree geograficamente molto distanti.

Il ruolo strategico della matematica per lo sviluppo delle aree depresse del pianeta è un dato ormai così acquisito da attirare investimenti di importanti società scientifiche come l’Unione Matematica Internazionale (IMU) e la London Mathematical Society che, in collaborazione con il prestigioso African Millennium Mathematics Science Initiative (AMMSI), con sede a Nairobi, già da qualche anno  ha lanciato il programma MARM (Mentoring African Research in Mathematics),  affidato sin dall’inizio all’autorevole e mirabile guida del professor Frank Neumann dell’Università di Leicester (UK). Lo schema funziona press’a poco così. Il consorzio LMS-IMU-AMMSI pubblica un bando con cadenza annuale, raccogliendo la disponibilità di matematici professionisti attivi in tutto il mondo ad assumere la mentorship (come si direbbe in italiano? Google dice “tutoraggio”, ma non rende l’idea) di un dipartimento di matematica di uno stato africano tra queli indicati dal bando stesso. Il matematico designato, e assegnato ad una specifica realtà, ha dunque il compito  di agire in modo da innescare un circolo virtuoso, contribuendo a formare un clima di vivacità scientifica che, come si è detto, si può ottenere al prezzo di sole interminabili (e divertenti!) discussioni con gesso e lavagna, che possa perpetrarsi anche, e soprattutto, dopo il termine del mandato del mentor. Nell’ultimo round la London Mathematical Society ha selezionato la Namibia (responsabile locale: Martin Mugochi), il Senegal, l’Etiopia e la Costa D’Avorio (https://www.lms.ac.uk/grants/ mentoring-african-research-mathematics) quali beneficiari del programma. Il Progetto MARM ha inoltre sostenuto, con un contributo finanziario, l’organizzazione, da parte della  National Commission for Research, Science and Technology (NCRST), della  Prima Olimpiade di Matematica della Namibia, un primo piccolo passo per avviare giovani talenti a partecipare alle competizioni regionali e internazionali.

  Val la pena di ricordare che molte Fields Medals (come se fossero i premi Nobel per la matematica) sono state vinte da campioni alle olimpiadi internazionali di matematica.  Un esempio nostrano: Alessio Figalli nel 2018, che vinse le Olimpiadi di Matematica al termine della sua formazione classica in un rinomato liceo di Roma.

Per rafforzare e consolidare i buoni risultati ottenuti con l’implementazione delle Olimpiadi di matematica è bene ricordare, argomento sul quale si ritornerà più avanti, che il 24 Ottobre prossimo si aprirà ufficialmente la prima edizione della Scuola Internazionale Primaverile di Matematica della Namibia, NAISSMA2022, sotto l’egida della London Mathematical Society, del Politecnico e dell’Università di Torino,  dello stesso NCRST e con l’Alto Patrocinio dell’Ambasciata d’Italia a Pretoria (competente anche per  il territorio namibiano), la quale garantirà la sua presenza con la partecipazione del Professor Pierguido Sarti, astrofisico, e Addetto Scientifico presso la Rappresentanza Diplomatica. Del resto l’appoggio diplomatico non è nuovo, poiché già si era materializzato nel corso del primo meeting MARM-NARM (From Mentoring to Networking African Researchers in Mathematics), che al saluto augurale del Primo Segretario di legazione Giulia Casagrande ha aggiunto il  mirabile intervento “International Cooperation. The Italian Way” del professor Pierguido Sarti, una specie di suggeritore occulto che ha sollecitato l’organizzazione e realizzazione diuna scuola internazionale di matematica in Namibia, con forte forte coinvolgimento europeo. NAISSMA2022, appunto.

La rappresentanza diplomatica, confermando la propria sensibilità e lungimiranza  e incarnando nei fatti lo spirito di cooperazione, ha erogato un sostanzioso contributo per l’acquisto dei premi (tablets, libri) da assegnare ai giovani vincitori delle Olimpiadi. Inoltre, quasi come per apporre un  sigillo  all’iniziativa, la cerimonia di premiazione ha visto la convinta ed entusiastica partecipazione della Vice Ambasciatrice Silvia Marrara che, a conclusione del suo intervento (il cui integrale può essere ascoltato attraverso la registrazione della diretta facebook dell’evento), ha affermato che: “In a world that is more and more looking at the new frontiers of the artificial intelligence, let me however to remind you that everything starts with human intelligence and that math sciences are the food for our next generation thoughts. Finally to conclude I would love  to mention Leonardo da Vinci, who said: “The one that fall in love with practice without science are like captains of a ship without a compass. They will never be sure of where they are going”.

Parole certamente opportune, otreché appropriate, per concludere questo articolo.  Non solo in quanto, provenendo da un non matematico, sono esenti da possibili (ma innocui) conflitti di interesse, ma anche perché, allo stesso tempo, fungono da suggerimento autorevole, ancorché  indiretto e magari non intenzionale,  per  future politiche scientifiche che possano consentire al nostro Sistema Paese di non rimanere troppo indietro in tempi resi incerti da una possibile prolungata austerità.

Letterio Gatto

Progetto MARM NAMIBIA

https://www.marm-lms.unam-namibia.org/

twitter.com/@unammarm1

facebook.com/@unammarm1

Anyone who believes in a substantial unity of the culture cannot avoid to refuse as definitely artificial the opposition of (the so called) sciences to (the so called) humanities. The sciences are human and we contend that humanities can be studied through the methods of the most rigorous sciences. Believing that they are split by a wall, unavoidably leads to big disasters: people may turn unaware that the culture is one crucial key to improve the general wellness.

The purpose of this short note is not to provide theoretical arguments to investigate a question that is  probably as old as the appearance of the rational thought, but to support our firm trusting in the unity of the culture, through a couple of relevant examples. They will be borrowed from topology, one of the purest branches among pure mathematics, which is in turn the most exact among exact sciences. This will show, the author thinks, an unsuspected link between pure maths and theology. Yes theology!  Topology¹ is concerned with the art of shaping the space,  by studying the properties that remain untouched under continuous deformations. It is also known as the rubber sheet geometry.

Continuous deformation of a sphere to an “ovoid”

Theology, instead, is concerned with God, an almighty being Who has designed the universe according to almighty wishes but could have drawn it in a different way. The question is: with  how much freedom?

This is the right moment to mention the following couple of (physical?) facts. The First. On the surface of the Earth, at each given instant time, there is a pair of antipodal points with the same temperature and the same atmospherical pressure, i.e. at least two points, at the antipodes, with exactly the same weather. The second.  On the Earth surface, crossed by winds, there are always  quiet spots, namely points where the winds do not blow. Are these Nature’s law? Is it possible to imagine a Universe, or many universes (pluriverses?) where such laws do not hold for some planet?

Let us begin to cope with the first “meteorological” situation. In the obscure times of the Second World War, two poolish mathematicians, Karol Borsuk (1905-1982) and Stanislaw Ulam  (1909-1984, who also participated to the famous Manhattan project, devoted to the realization of the first atomic bomb), stated and proved an astonishing theorem. Recall: a theorem is a proposition whose truth must be validated by a rigorous proof.  In a simplified form,  Borsuk and Ulam’s result² says that every continuous function from the sphere to the plane takes the same value at least to one pair of antipodal points.  Let us explain to non-mathematicians what this does mean. Recall: two points of a spherical surface are said to be antipodal if they are diametrically opposed (one usually says that Italy and Australia are antipodal). Since the points of a plane can be labeled by pairs of real numbers, as depicted in the figure below, to give a function from the sphere to the plane is the same as labeling all the points of its surface by a pair of real numbers (that supplies the correspondence to a point of the plane).

For better explanation, it can be helpful going directly to the example. Consider the “function” associating to each point of the Earth, thought of as a physical model of a sphere, its pressure and its temperature. This is a function with values in a plane, because to each point of the sphere one is attaching a point in the “pressure – temperature” diagram. What does it mean that such a function is continuous? Roughly speaking, it means that if a point of the sphere has a given pressure and temperature (P,T), there are neighboring points whose labeling pairs (pressure, temperature) differ from the given data as little as one desires. Putting otherwise, although not completely correct, it means that if we move in a close neighbourhood of the point, there is a little variation of the pressure and of the temperature.

                                   Two antipodal points on a Sphere’s surface           The point represented by the bullet has been labeled with (2,1)

The issue is now: how to guarantee that pressure and temperature vary continuously with the position? This is not proven. This is Faith. Better, it is metaphysics. We trust  that (physicists do) basing upon experimental (but possibly failing) evidence. And assuming continuity,  Borsuk and Ulam ensure us that there MUST be two antipodal points on the sphere with the same  pressure and temperature, just because the function {point of the sphere}—>(its temperature, its pressure) is continuous by hypothesis. In other words: there are two antipodal points on the Earth with the same weather!

The second phenomenon we want to mention is that of the eye(s) of the storm(s), the point, or the points, on the Earth surface where there is no wind blowing. The existence of such spots  is a necessary consequence of the topology of the sphere as well. It is related with the following classical purely topological theorem: there is no tangent vector field on a sphere that does not vanish to at least one point (indeed, it vanishes to at least two points). What is a tangent vector field? The wind is an example. But you can also think to the hairs of the head. You can think of each hair as if it were a model of a vector (in college physics books they are usually represented by arrows, having a magnitude and a verse, used to describe forces or physical fields). So, you can think of the set of all hairs on your head as a model of a vector field. If you are hair bumped, then the vector field is not tangent. But if you comb your hairs you may  turn them into a tangent vector field

                                                                       A non tangent vector field                             A tangent vector field: can you distinguish an eye of the storm?

The aforementioned theorem then claims that the sphere is not a combable surface: imagine to be given a radial field of “hairs”, one at each point of a sphere. The theorem demands that to completely  comb it,  you must remove at least two hairs (points where the “hairs” tangent vector field vanishes). As a consequence,  any continuous wind vector field on a sphere’s surface, MUST vanish to at least two (may be more, but at least two) points where the wind does not blow. These are the eyes of storms.

                                                                                      Combing a sphere                                                            Eye of the storm

In conclusion, it seems that mathematics is able to impose laws to Nature, like e.g the same weather at two antipodal points or, as seen, the eyes of storms irrevocably destined to be attached to a winds’ field. Have they to be considered as Nature’s laws? Or they rather are necessary ones, unavoidable ones? The reader may object that the Earth is not a perfect sphere. But that does not matter, you know. This is topology, whose properties hold for all surfaces that can be continuously deformed one into another. If X can be continuously changed into a sphere (imaging it made of a sort of magic rubber that can be deformed and shape at anyone own wish) then a) a plane valued continuous function on X must take the same values to at least one pair of distinct points and b) any tangent vector field must vanish to at least two points (you cannot comb it completely).

To come to the theological impact we ask ourselves: are the aforementioned results prescribing any kind of limitation to the power of God? Think a little bit about. The discussed theorems are saying us that God (or the Nature) can create universes where temperature and pressure, or wind blowing, may not vary continuously on a sphere’s surface. That is possible, of course. However, if continuity is preserved and/or demanded, He cannot prevent that at least one pair of antipodal points take the same temperature and pressure and He cannot avoid a wind having his own eye of storm.  Mathematics is thus prescribing, in these occurrences, laws concerning not the Pangloss’³ best possible World, but rather a rule that must be necessarily obeyed in all the possible worlds. As we have argued,  the necessity of mathematics constrains the possibilities of Physics. This is quite striking.

Eventually, we believe that who does not stop a little bit to taste the magic flavor of  theorems like that of Borsuk and Ulam, to reflect about the necessaries laws of an over-Nature that sometimes overcomes the empirical step of the scientific investigation, is going to loose the human, very human, too human side of the Science. He will loose all the fun which is the true joy of the scientific research. “There are more things in Heaven and Earth, Horatio, than are dreamt of in your philosophy”⁴. Nowadays, we could phrase Hamlet’s warning as “there are many more interesting questions than boring anwers”. Not just general, negligible questions, but queries that are relevant to our everyday life, to our beliefs, to our faiths, to our dreams.

Bibliographical Notes

¹ For a general elementary and substantially self contained introduction to topology, see the book by Wallace, An Introduction to Algebraic Topology (Dover Books on Mathematics).

² The theorem of Borsuk and Ulam is one of the most popular theorems in topology. Several readable accounts have been published. See e.g. the book by Massey, A basic course in Algebraic topology, Springer GTM 127.

³ Voltaire, Candide.

⁴ W. Shakespeare, Hamlet, Act 1, Scene 5.